Sine, cosine and tangent

At school we first learn how to measure angles and how to add and subtract angles. Later on comes the idea of sine, cosine and tangent. There is a question that is left unanswered until we learn the definitions of sine, cosine and tangent.

The question is: we know that a ramp can be harder to walk on according to how much inclined it is, with flat being zero angle and 90° the hardest. Is there a relationship of how many units we walk forwards and how many units we go up if we are walking over a ramp with an angle greater than zero? This is exactly what sine, cosine and tangent are. Ratios that relate angles to sides of a triangle such that we have the answer for the previous mentioned question.

Note: angles do not make sense if we do not have at least two dimensions.

Caution: we always define tangent, cosine and sine with a right triangle. If the triangle does not have a right angle we cannot state that the proportions hold. In fact, they don't.

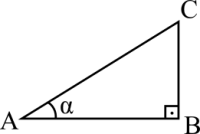AB = run = base BC = rise = height AC = ramp = hypotenuse

In Euclidean geometry the sum of all internal angles must be equal to 180°. With the right triangle having one angle being 90°, the sum of the other two must be 90°. This explains why we never have to memorize rules or properties beyond 90°, because we don't need to.

[math]\displaystyle{ \sin(\alpha) = \frac{BC}{AC} }[/math]. It means how many units we are going up in respect to how many units we are walking forwards over the ramp. What happens if the angle is zero? Then we are not going up no matter how many units we go forwards. This explains [math]\displaystyle{ \sin(0^{\text{o}}) = 0 }[/math]. What happens if the angle is 90°? Then we have a 1:1 ratio, for each unit of the triangle's height we walk over the ramp by the same units. This explains [math]\displaystyle{ \sin(90^{\text{o}}) = 1 }[/math].

[math]\displaystyle{ \cos(\alpha) = \frac{AB}{AC} }[/math]. It means how many units we are going forwards in respect to how many units we are walking up over the ramp. What happens if the angle is zero? Then we have a 1:1 ratio, for each unit of the triangle's run we walk over the ramp by the same units. This explains [math]\displaystyle{ \cos(0^{\text{o}}) = 1 }[/math]. What happens if the angle is 90°? Then we are not moving forwards while going up. This explains [math]\displaystyle{ \cos(90^{\text{o}}) = 0 }[/math].

[math]\displaystyle{ \tan(\alpha) = \frac{BC}{AB} }[/math]. It means how many units we are going up in respect to how many units we are going forwards. What happens if the angle is 90°? The tangent doesn't exist. Think about a circle on the plane and a point in its perimeter, a perpendicular line (on the same plane) cannot be tangent to that point, it's impossible. What happens if the angle is 45°? Then we have a 1:1 ratio, for each unit we go forwards we also go up by the same unit. This explains [math]\displaystyle{ \tan(45^{\text{o}}) = 1 }[/math]. What happens if the angle is zero? Then we are not going up for each unit we walk forwards. It's the same as being parallel.

It's pretty easy to confuse sine with cosine and, for the same reason, invert the two and confuse the tangent too. At school there are some puns that people make to memorize that the horizontal axis is x and the vertical is y. If you look at the triangle above, sine of the angle is always the opposite side divided by the ramp's length. Cosine being the adjacent side divided by the ramp's length. Tangent being height / base. I think this is a way to memorize that sine is always related to the height first, whereas cosine is the base first. Therefore, the vertical / horizontal ratio is the tangent.

There are numerous practical examples that teachers use to demonstrate trigonometry in the real world: length of shadows, height of buildings, solar clocks, etc. Physics has many examples such as stone skipping or reflection of light on the surface of water. My goal here is not to explain each and every example.

Viewing sine, cosine and tangent as percentages

(not to scale)

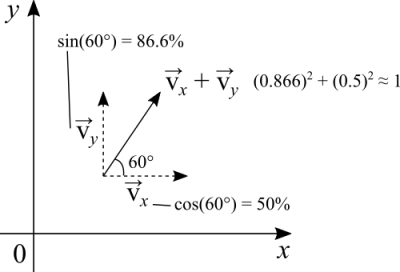
Another way to interpret the ratios is a percentage, because every ratio is a percentage. Sine, cosine and tangent assume values between 0 and 1, with the values between 0 and -1 being reflections. Let's see the above vector as displacement, with a length of 1 to make it easier. We have that:

[math]\displaystyle{ \overrightarrow{v} \ \ = (0.866, 0.5) }[/math]
[math]\displaystyle{ \overrightarrow{v}_x = (0.866, 0) }[/math]
[math]\displaystyle{ \overrightarrow{v}_y = (0, 0.5) }[/math]

If we have a scalar quantity such as time, mass or money we can divide it in smaller parts such as 50% + 50% = 100%. With vectors we cannot have the same interpretation with velocity, kinetic energy or displacement. Recall that with sine, cosine and tangent we are calculating a ratio that is length / length. When we do a quotient in physics with quantities that have the same units, such as m/s by m/s or Newton by Newton. The unit cancels out. In the diagram above, 86.6% means that if we take the vertical magnitude and divide by the magnitude of the hypotenuse, we find out that the vertical component's magnitude is 86.6% of the hypotenuse. Another angle, 45°. Tangent of 45° is 1, which translates to 100%. 100% of what? Tangent, by definition, is height / base of the right triangle. 100% in the case of vectors mean that one component has the same magnitude as the other. It doesn't mean "total magnitude" of whatever vector we have.